Future Value Calculation and the Rule of 72

Getting down and dirty with the CAGR formula

The Basics

Let’s say you have an asset worth $100 now, and you want to find out what it’s value will be in 5 years if it grows at a rate of 10% per annum and interest is compounded.
The equation is simple. The asset’s future value (FV) and present value (PV) are related as follows:
$$FV = PV\times(1+i)^n$$
where i is the rate of interest and n is the number of periods. In our example:

$$FV = $100\times(1+0.10)^5 = $161.051$$

Here the total growth over the 5 years FV/PV is 161.051/100 = 1.61051 . This gives us the total cumulative return r.

$${FV \over PV} = (1+i)^n = 1+r$$

Or expressed in percentage terms:

$$r = [(1+i)^n - 1] \times 100$$
(equation A).
In our example, (1.61051-1) x 100 = 61.051%.
If we rewrite the future value calculation to solve for i:
$$i = \left[FV \over PV\right] ^{1/n} - 1$$
(equation B),
then substituting in for r and in percentage terms:
$$i = [ (1+r)^{1/n} - 1 ] \times 100$$
(equation C).
Note that equations marked A and C are quite symmetrical. The form of Equation B is termed the compound annual growth rate (CAGR).
If we wanted to solve for n, the formula becomes more involved:
$$n = {log(FV) - log(PV) \over log(1+i)}$$
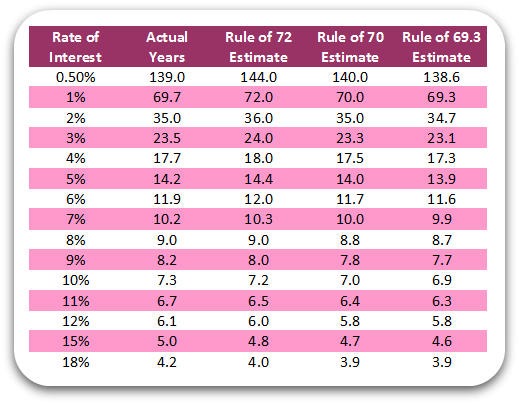
Typically we can use the “Rule of 72” to derive a quick approximation for how long it will take to double an investment value.


The Rule of 72

The Rule of 72 is a very simple way of illustrating the growth potential of compound interest. The rule says simply this:
$${72 \over i} \approx n$$
where i is the interest rate in percentage, and n is the number of time periods needed to double the principal.
For example, say an investment fund grows at 12% average annual rate. According to the rule of 72, if money were invested in this fund, then it would double every 6 years (72/12 = 6).
However, the above Rule of 72 merely gives an approximation of the time needed to retain an investment before it doubles in value. The accurate calculation is as follows:
$$n = {ln2 \over ln(1+i)}$$

Alternatively, the Rule of 70, or 69.3 is used for more accuracy at lower interest rates.

rule-of-72















Popular Posts

How to Read a Fund Fact-Sheet

Image
Has your adviser given you a factsheet on a recommended fund, and you need to understand what the terms mean? If so, read on … The fund factsheet is an essential document for the private investor. Unfortunately, the information contained in a factsheet - and the way it is presented - is not controlled in the same way as it is in a fund prospectus (a prospectus is a legal document). In essence a factsheet is marketing literature. Only a tiny fraction of investors ever read the formal prospectus for a fund, that’s why it’s especially important to be able to read a fund factsheet correctly, and if necessary to read between the lines. Key Contents Of A Factsheet Most fund factsheets will contain some or all of the following: Fund Name Many funds are commonly referred to by a short or abbreviated version of the full fund name. Hence it’s important to make use of the identifying codes (see below). Fund Management Company & Domicile The fund management company is structured and reg...
Read more

Understanding Compound and Annualised Growth

Image
Across fund manager houses, there is little standardisation of how performance figures are presented on fact sheets. Percentage returns are usually presented as either (i) discrete (or calendar) annual, (ii) cumulative (for example a total return figure for 3 years or 5 years) or (iii) annualised (the equivalent annualised return for a period such as 3 or 5 years, sometimes called compound annual return). This tutorial shows you how to convert from discrete annual performance figures, to cumulative or annualised figures, over a period of your choice. As an example, we’ll look at some USD fund performance data presented in an October 2010 factsheet. The performance data are presented to us in a very clear way, but may not be the complete picture we are looking for. The table directly above shows the discrete annual returns - ie. net of fees the fund delivered 7.46% in 2009, -4.90% in 2008, 9.82% in 2007, etc. But let’s suppose you ask yourself (or your advisor) “What was the total growt...
Read more

Tax Free Savings Plans for Australians?

Image
Question: How can returning Australians (and expats moving to Australia) make MASSIVE tax savings by using offshore investment bonds and investment-linked life policy wrappers? Answer: Set it up while you’re an expat working abroad, and keep it running at least ten years (even if you go back home) for maximum tax benefits. (Note: this article is relevant to expatriate Australians working in Singapore and other overseas locations, as well as other nationalities who may be moving to Australia in the future.) Click to download a copy of this information in PDF format. Background - Repeal of Foreign Investment Fund legislation For several years the Australian Tax Office (ATO) had very defined, and perhaps harsh, rules for how foreign insurance bonds were treated in respect of capital gains for tax residents in Australia. In effect, these tax wrappers were treated in a ‘look through’ manner and holders were liable for tax on actual gains made or at a deemed rate set by the authorities...
Read more

British Expat? Here are the Huge UK Tax Benefits of an Offshore Investment Plan...

Image
Would you like to maximise the tax planning benefits of being a British expat abroad? Read on for a summary of some key reliefs and allowances available from the HMRC tax man… (Note: this article is relevant to British expatriates working in Singapore and other overseas locations, as well as other nationalities who may be moving to UK in the future.) Click to download this information in PDF format. Summary of Tax Benefits of an Offshore Investment Plan Under HMRC tax rules for ‘ foreign ’ investment bonds and investment-linked life policies have additional tax planning benefits compared with their domestic equivalents. The purpose of this article is to briefly highlight the most important. The content is not intended to be specific tax advice of any kind, and rules can change at any time. Everyone’s tax situation is unique and you should consult a qualified tax accountant regarding your own circumstances. 5% withdrawal allowance Time apportionment reduction Top slicing relief ...
Read more

Key Interest Rate Definitions

Image
Definitions of the various types of interest rate Central bank rate - is the one most generally referred to in the financial press when they talk about the ‘bank rate’ or ‘interest rate’ without further specification (except for the USA, see below). Set by policymakers and usually the key rate upon which others are based. Also called the ‘base rate’ in the UK, ‘cash rate’ in Australia and New Zealand, and ‘discount rate’ in the USA. It’s the rate at which the central bank lends money overnight to commercial banks. Federal funds rate - specifically in the USA, policymakers at the Federal Reserve use a slightly different mechanism for influencing the nation’s interest rates. The federal funds rate is the overnight rate at which banks lend reserve balances to each other. The Federal Reserve Open Market Committee (FOMC) sets a target for this rate and implements monetary policy through ‘open market operations’ - buying or selling government securities (Treasury bills and bonds) in the op...
Read more

Should UK Expats Pay National Insurance Contributions?

Image
I live outside the UK - should I make voluntary National Insurance contributions? The decision on whether to make voluntary NI contributions depends on your own specific circumstances. National Insurance contributions for British expats? First a quick reminder. National Insurance (NI) contributions go principally to fund the National Health Service (NHS), the state pension, unemployment benefits, and sickness and disability allowances. Anyone employed in the UK will have an NI number, and make NI contributions via PAYE (pay-as-you-earn) deduction from salary. These are known Class 1 NI contributions (NICs). If you’re self-employed in the UK, you pay Class 2 NICs at a flat weekly rate, and annually Class 4 NICs based on taxable profits. If you are working abroad for up to two years and for a UK-based employer, you will likely be required to continue paying Class 1 NICs.  Alternatively, you can choose to pay voluntary contributions, Class 2 or Class 3. Depending on your circumstances...
Read more

Mobile Phone Screens Are Getting SMALLER!

Image
If you bought a “6-inch” screen phone this year, it will have a SMALLER screen than the “6-inch” screen phone you bought two years ago.  Here’s why. Call me old-fashioned (or just old), but I spend a lot of time reading stuff – articles, charts, and data – on my phone.  And I mean actually reading it – not ‘skimming’ through an article while my thumb is continually scrolling the page upwards. A ‘Full HD’ screen is 1920 x 1080 pixels – which is nice for movies, and gives an eye-friendly ‘book page’ ratio of 16:9.   This has been the standard screen ratio of mobile phones for several years, up to the last year or so, when suddenly manufacturers have switched to a 18:9 ratio  (which is 2:1 by the way… no idea why they call it 18:9, duh).   For me, 16:9 is perfect for reading text.  2:1 isn’t – it’s too thin and narrow.   Supposedly, manufacturers have switched to this format because phones with skinnier screens fit into our pockets better,...
Read more

Investment Outlook For 2018

Image
After a nervous start to 2017 for investors, growth continued and the year ended on a higher note than most expected.  As we enter 2018, it’s a good time to survey the global economy and decide if our asset allocation is still appropriate. In this article I aim to address these straightforward questions: Will there be a recession in 2018? How cautious should I be in my investment approach? Where are the investment opportunities for the year ahead? What asset classes should I overweight or underweight in my portfolio? I will also reveal why an ox weight-guessing competition, held in 1906 at the West of England Fat Stock and Poultry Exhibition, helps us derive more useful information than individual leading economists do about the risk of imminent recession. This is good stuff, you really should read on! Please share this article! >> Review of Key Economic Reports Remembering the First Law of Economists , it’s a good idea to conduct a broad survey of what ...
Read more

I Don’t Need A Financial Adviser!

Image
“I don’t need a financial adviser - I can do everything myself.” If you hear yourself thinking this - great! Because it’s almost certainly true. Every single one of my clients has the intelligence and common sense to do what I do.  Though in general, they don’t have the time to commit to this important subject. Often I hear this phrase from people who’ve never worked with a professional adviser before, and never sat down with one to find out what’s on offer. I think maybe it could be down to a misunderstanding of the role. As A Manager, Do You Have Problems Delegating? Pretty much every senior manager I know has earned his or her position by being good, often excellent, in previous roles. That means that the Sales Director was probably a great salesman before. The CFO was probably a great management accountant. The Technical Director was a great systems analyst. The Marketing Director could probably still turn out great advertising copy. In fact, each could excel doing the job of e...
Read more